Physics Notes - Herong's Tutorial Notes - v3.24, by Herong Yang
Length Contraction Formula and Lorentz Factor
This section provides steps to derive the length contraction formula expressed with the Lorentz factor, based a thought experiment and the time dilation formula.
From the previous section, we collected two sets of observations from two reference frames:
L = c*T/2 (L.6) - Carriage length observed by Amy L' = (1-(v/c)**2)*c*T'/2 (L.21) - Carriage length observed by Bob
Now if we agree with the time dilation formula discussed earlier, we can derive the length contraction formula as below:
T' = T/sqrt(1-(v/c)**2) (L.22) - Time dilation formula gamma = 1/sqrt(1-(v/c)**2) (L.23) - Set "gamma" as Lorentz factor T' = gamma*T (L.24) - L.23 in "gamma" format L' = (1/gamma**2)*c*T'/2 (L.25) - L.21 in "gamma" format L' = (1/gamma**2)*c*(gamma*T)/2 (L.26) - Merge L.24 into L.25 L' = (1/gamma)*c*T/2 (L.27) - Simplified L.26 L' = (1/gamma)*L (L.28) - Merge L.6 into L.27 # Length contraction formula
Congratulations, we have derived the length contraction formula! The formula tells us that the carriage is observed to be shorter by Bob on the ground than what observed by Amy moving with the carriage, because 1/gamma < 1.
The length contraction formula can also be expressed in mathematical format as:
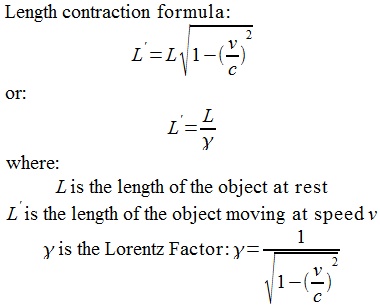
Table of Contents
Introduction of Frame of Reference
Introduction of Special Relativity
Time Dilation in Special Relativity
►Length Contraction in Special Relativity
Length Contraction - Moving Object Is Shorter
Demonstration of Length Contraction
►Length Contraction Formula and Lorentz Factor
Reciprocity of Length Contraction
The Relativity of Simultaneity
Minkowski Spacetime and Diagrams
Introduction of Generalized Coordinates